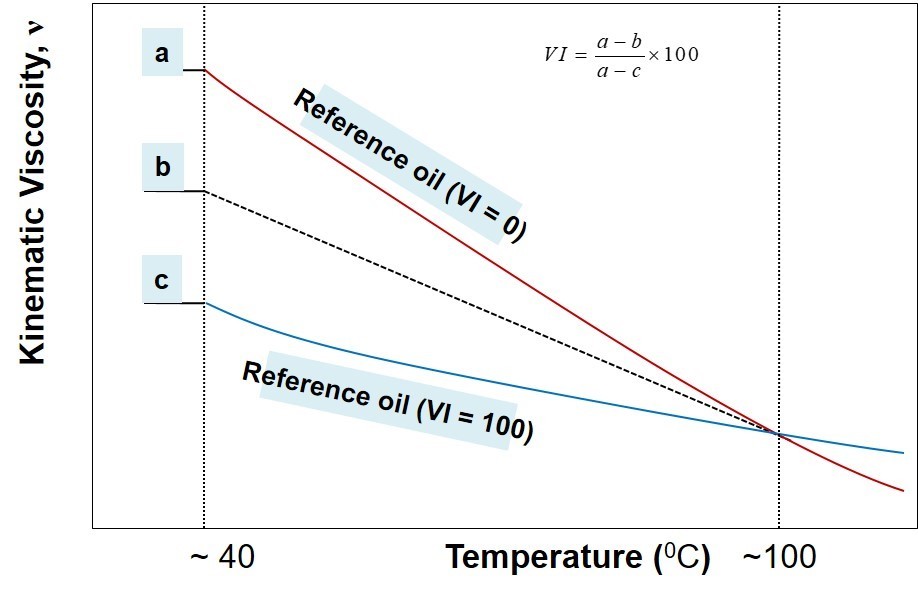
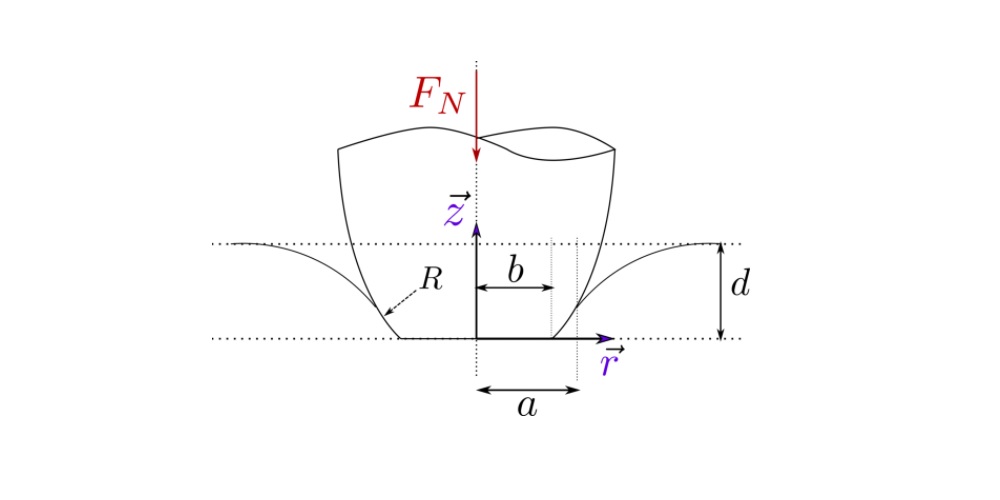
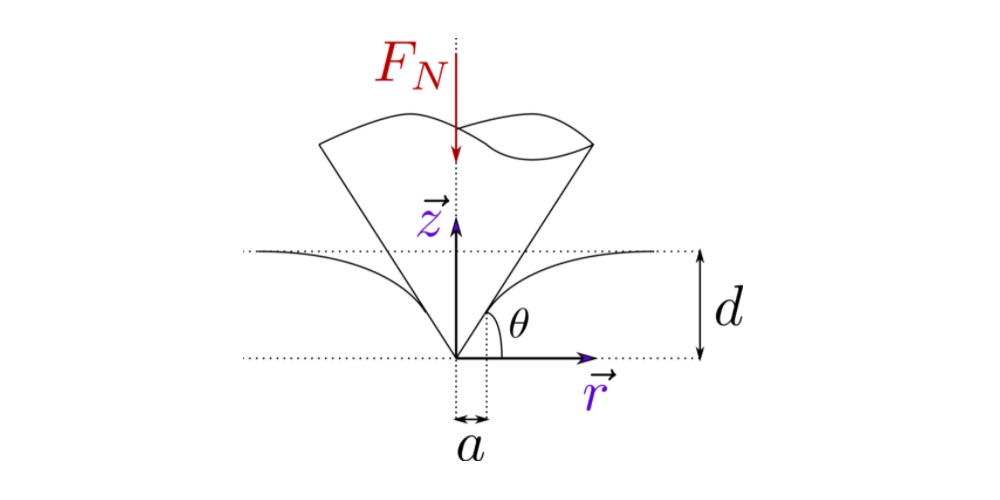
Administration of the project
Indentation by a Flat Elliptical Punch: Online Calculator
06.11.2018

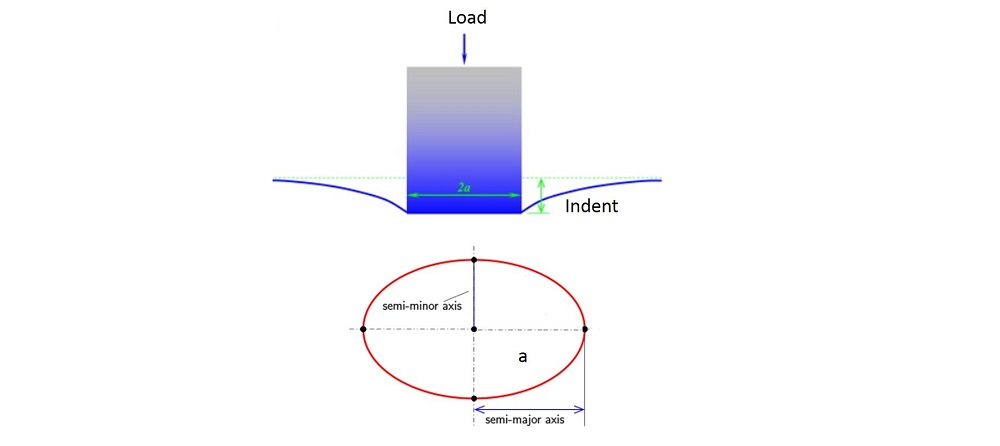
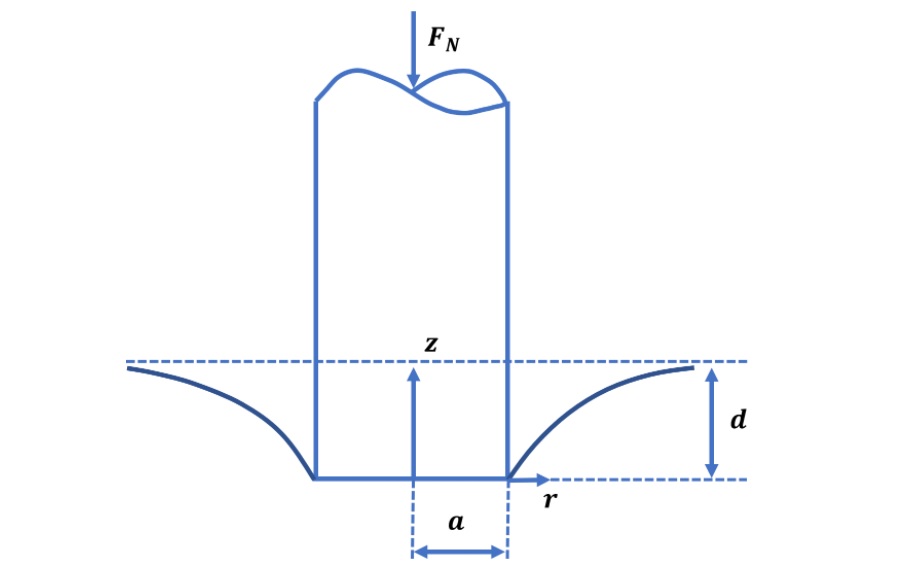
This is a simple calculator for an elastic indentation by a flat punch.
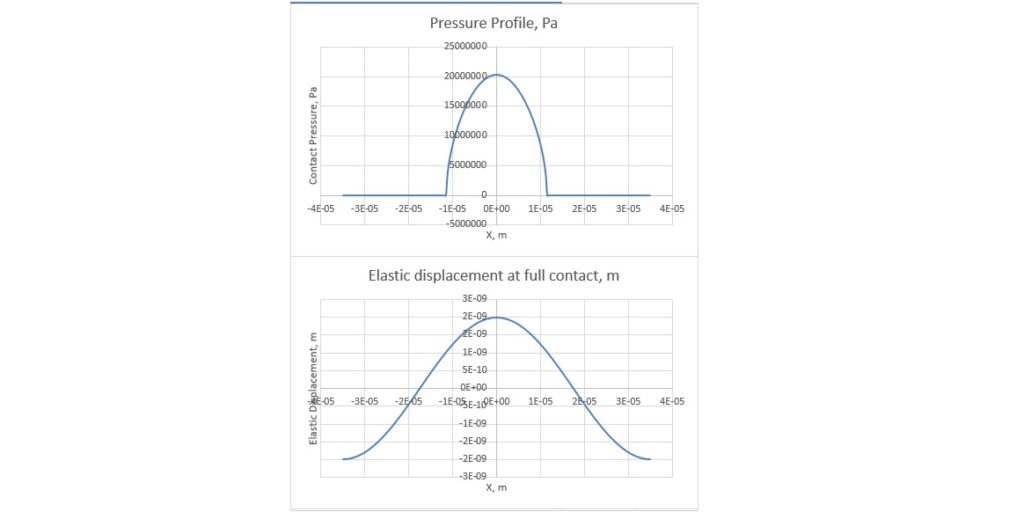
Pressure profile can be calculated as follows:

where ![]() ,
, ![]() being semi-major axis and
being semi-major axis and ![]() being semi-minor axis,
being semi-minor axis, ![]() – normal load.
– normal load.
Indentation depth is calculated using following equation:
![]() , where
, where ![]() is the complete elliptical integral of the first kind,
is the complete elliptical integral of the first kind,  is eccentricity,
is eccentricity, ![]() is the reduced elastic modulus,
is the reduced elastic modulus, ![]() .
.
Equations are taken from [1].
Note: the elliptic integral of the first kind is required to calculate the indent value. An approximate formula is used here (http://www.exstrom.com/math/elliptic/ellipint.html).