Administration of the project
Rigid Flat Punch on Flat: Online Calculator
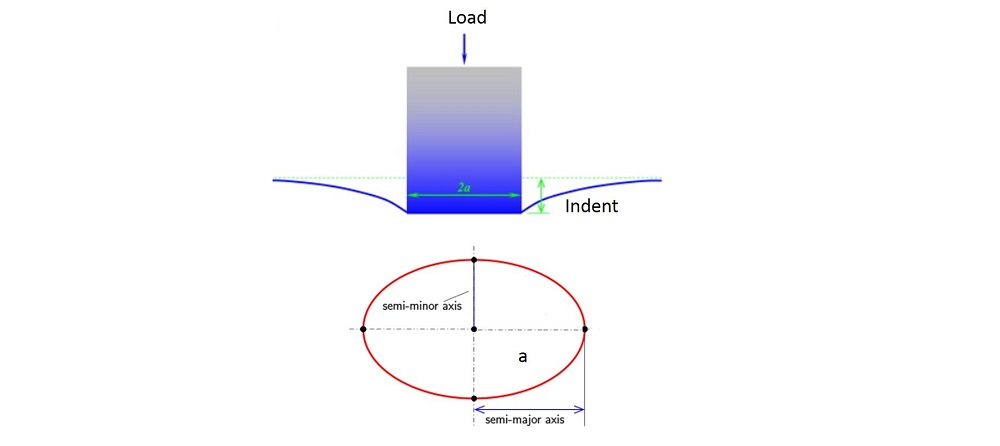
Indentation by a Rigid Flat Punch
This calculator allows calculating pressure ![]() , displacement (elastic deformation)
, displacement (elastic deformation) ![]() , stiffness
, stiffness ![]() , average pressure
, average pressure ![]() and resultant force
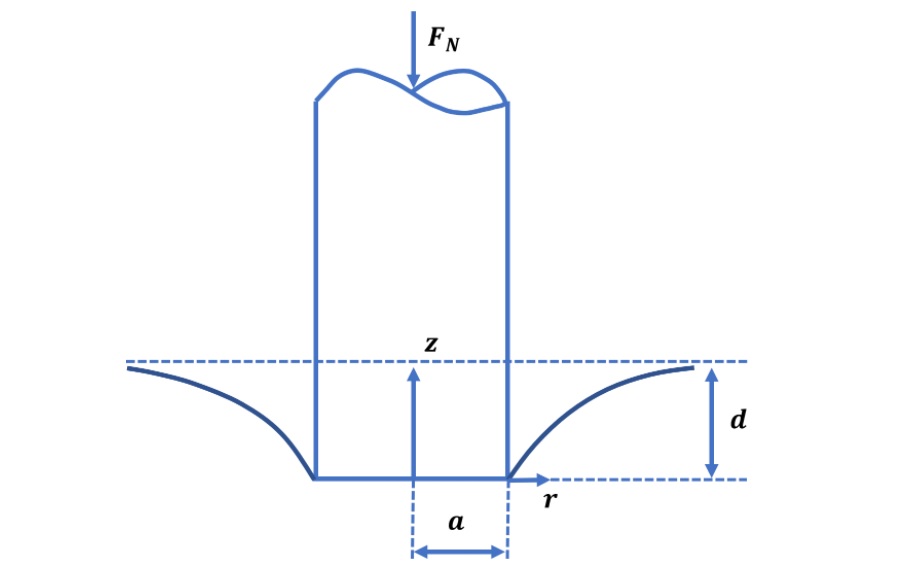
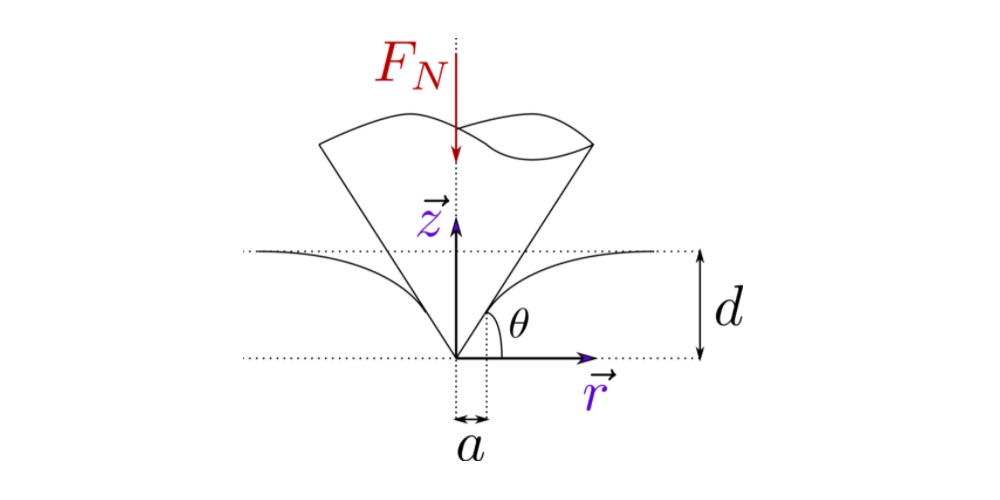
and resultant force ![]() for the case of indentation of an elastic half-space by a rigid cylindrical flat indenter. In this case, the intender is rigid and of cylindrical shape. The substrate is elastic, the geometric parameters
for the case of indentation of an elastic half-space by a rigid cylindrical flat indenter. In this case, the intender is rigid and of cylindrical shape. The substrate is elastic, the geometric parameters ![]() – rigid body displacement,
– rigid body displacement, ![]() – radius of the cylinder and material parameters
– radius of the cylinder and material parameters ![]() are the inputs (see the figure and definitions below). The stress distribution in the contact area and the displacements of the half-space outside the contact are:
are the inputs (see the figure and definitions below). The stress distribution in the contact area and the displacements of the half-space outside the contact are:
(1) 
where ![]() is the maximum displacement due to the deformation,
is the maximum displacement due to the deformation, ![]() is the radius of the punch and
is the radius of the punch and ![]() is the radial coordinate.
is the radial coordinate.
The contact stiffness can be calculated as follows:
(2) ![]()
Equations were taken from [1].
Definitions:
Poisson’s ratio ![]() dimensionless,
dimensionless,
Young’s modulus of elasticity ![]() , [Pa],
, [Pa],
Equivalent elastic constant  , [Pa],
, [Pa],
Normal load ![]() , [N]
, [N]
Take a look at other calculators: https://www.tribonet.org/calculators/.