Administration of the project
Truncated Paraboloid on Elastic Flat: Online Calculator
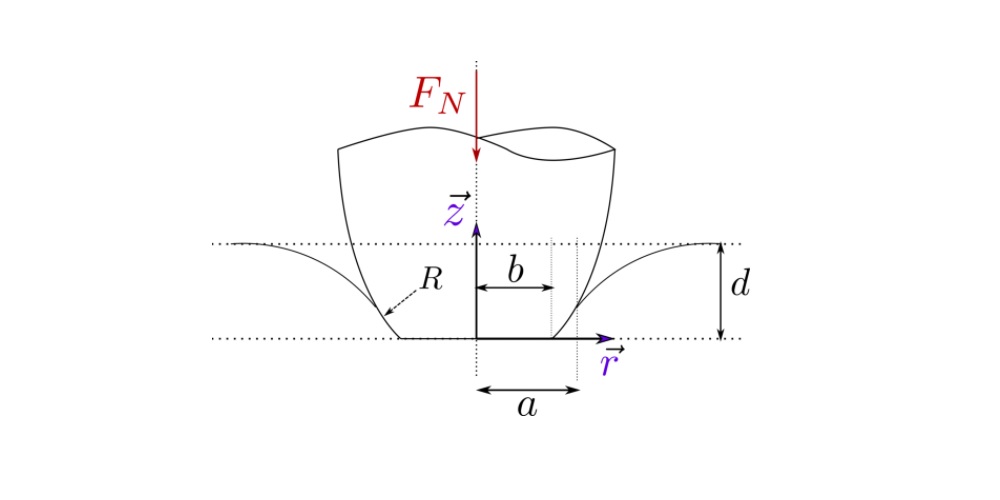
This calculator allows calculating pressure ![]() , displacement (elastic deformation)
, displacement (elastic deformation) ![]() , average pressure
, average pressure ![]() and resultant force
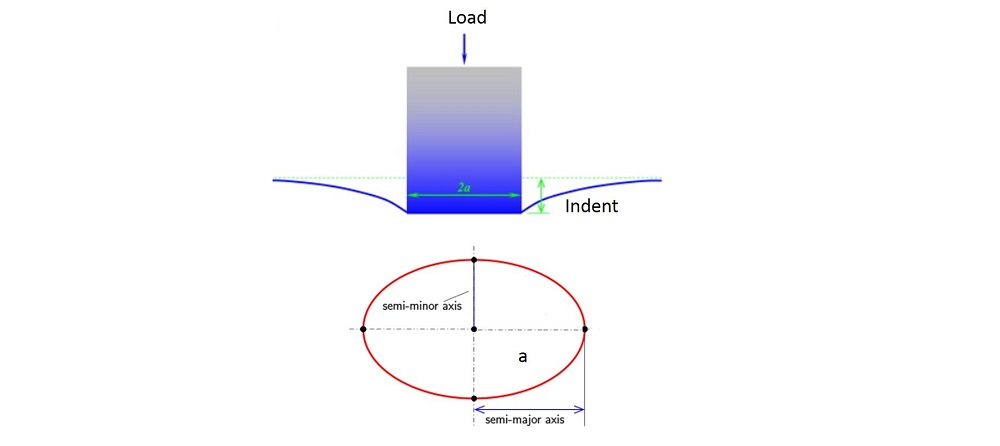
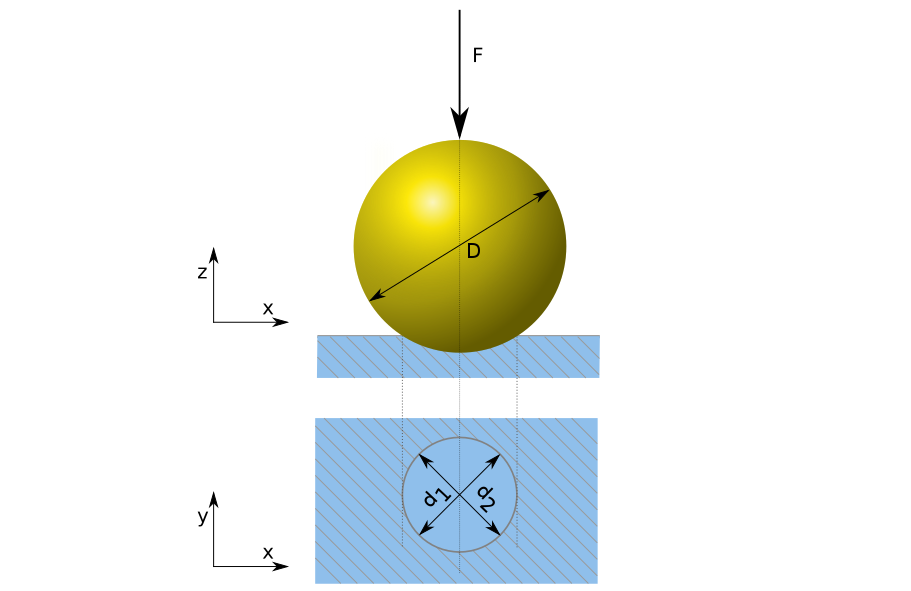
and resultant force ![]() for the case of indentation of elastic half-space by a rigid truncated paraboloid. The substrate is elastic, the geometric parameters,
for the case of indentation of elastic half-space by a rigid truncated paraboloid. The substrate is elastic, the geometric parameters, ![]() – radius of curvature,
– radius of curvature, ![]() – radius of the blunt end, indentation depth
– radius of the blunt end, indentation depth ![]() and material parameters
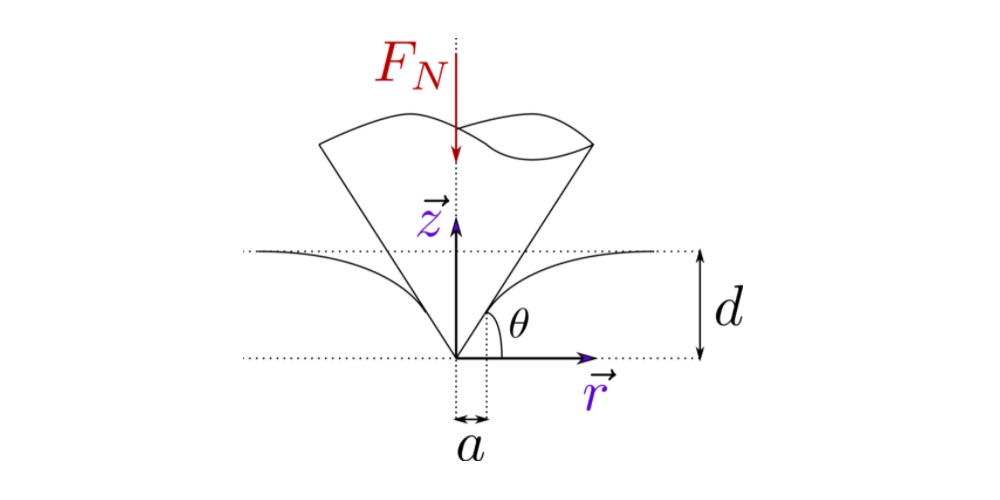
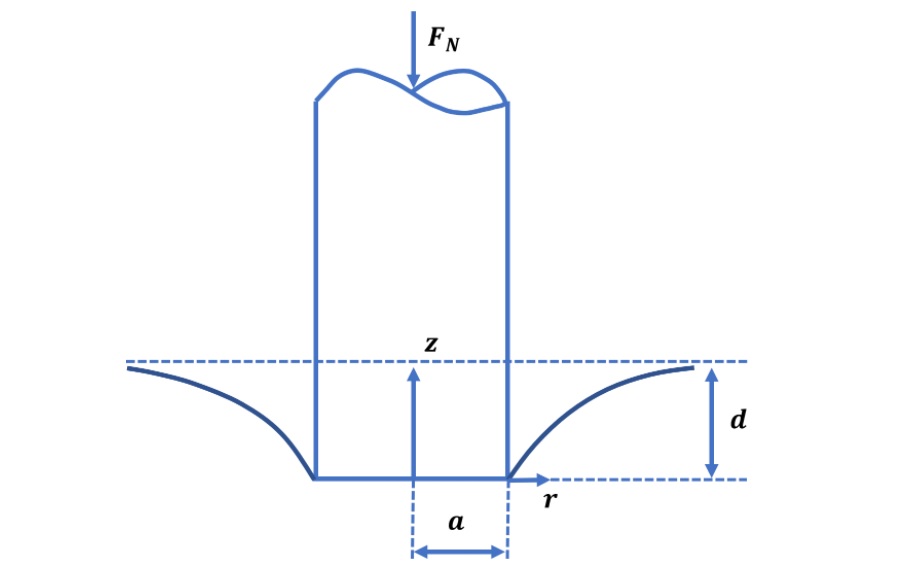
and material parameters ![]() are the inputs (see the figure and definitions below).
are the inputs (see the figure and definitions below).

A truncated paraboloid is characterized by the blunt radius ![]() that covers the base, the contact radius
that covers the base, the contact radius ![]() and the curvature
and the curvature ![]() . The intender is described by the profile:
. The intender is described by the profile:
(1) 
The contact radius ![]() can be deduced numerically using the relation that connects the geometrical parameters to each other:
can be deduced numerically using the relation that connects the geometrical parameters to each other:
(2) 
Other parameters: Load force ![]() , displacement
, displacement ![]() and the stress profile
and the stress profile ![]() are given by the following formulas:
are given by the following formulas:
(3) 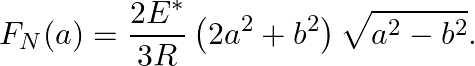
(4) 
(5) ![Rendered by QuickLaTeX.com \begin{align*} \omega(r;a) &= \frac{2a}{\pi R}\sqrt{a^2-b^2}\arcsin\left(\frac{a}{r}\right) \nonumber\\ &-\frac{1}{\pi R}\left[(r^2-b^2)\arcsin\left(\frac{\sqrt{a^2-b^2}}{\sqrt{r^2-b^2}}\right) - \sqrt{a^2-b^2}\sqrt{r^2-a^2}\right], r>a. \end{align*}](https://quicklatex.com/cache3/37/ql_74844671816fe7d8d0302d5e96f97737_l3.png)
Equations were taken from [1].
Definitions:
Poisson’s ratio ![]() dimensionless,
dimensionless,
Young’s modulus of elasticity ![]() , [Pa],
, [Pa],
Equivalent elastic constant  , [Pa],
, [Pa],
Normal load ![]() , [N]
, [N]